•     Particle Exchange Model of Forces
•     Barrier Penetration in Particle Exchange
•     Quantum Operators & Matrices
•     2-State & N-State Systems
•     Hyperfine Splitting & Spin-Spin Interactions
•     Electrons in Crystals & Semiconductors
•     Schrödinger’s Equation
•     Symmetry & Conservation Laws

An Ionized Hydrogen Molecule

We consider here a hydrogen molecule that has been ionized by the removal of one electron, leaving two protons to share the lone remaining electron. Let’s examine how this electron can be shared.

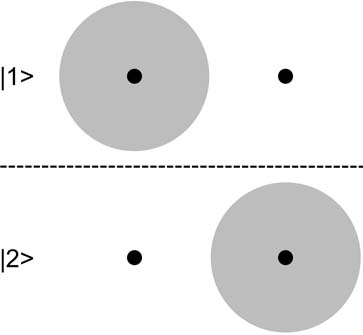
Two simple basis states present themselves: the electron could surround the left proton; or it could surround the right proton. These two states are symmetric and both effectively result in a neutral hydrogen atom with a lone proton nearby, as shown in Figure 13-1.

The energy required to remove an electron from a neutral hydrogen atom is 13.6 eV. On the atomic scale, 13.6 eV is substantial, about 6 times the energy of visible light.

For the electron of an ionized hydrogen molecule to jump between states |1> and |2> it would have to overcome the binding energy of one proton before falling into the potential well of the other proton. This is a bit like a golf ball spontaneously disappearing from one cup and reappearing in another. No golfer has ever seen this happen, because in classical physics such actions are impossible.

However, as we discovered in Chapter 9, barrier penetration does occur in the realm of quantum mechanics. Hence there is a non-zero amplitude H12 for the transition from |2> to |1>. Since 13.6 eV presents a large barrier, the amplitude H12 is small. By symmetry, states |1> and |2> must have the same energy (H11=H22) and the same amplitude to transition from one to the other (H12=H21).